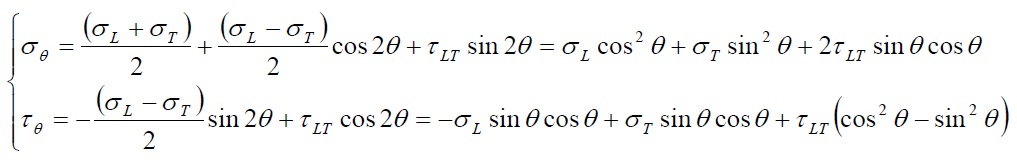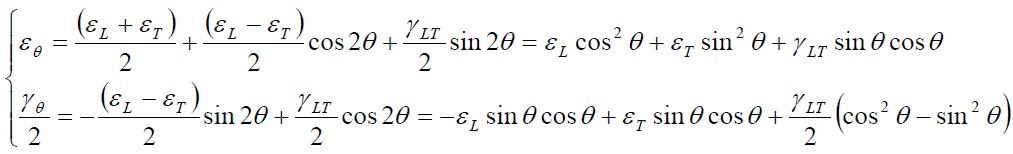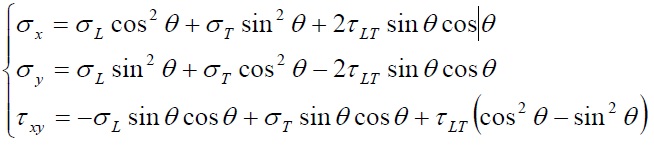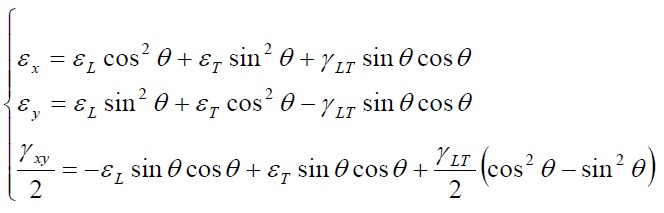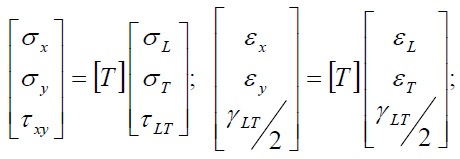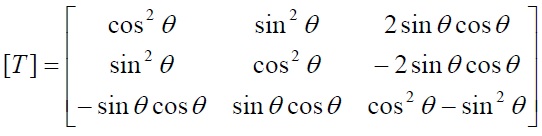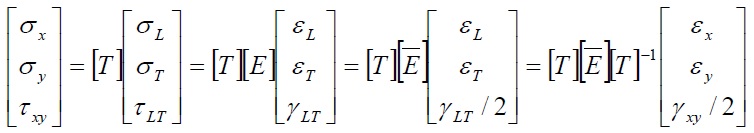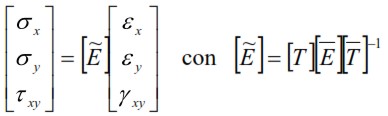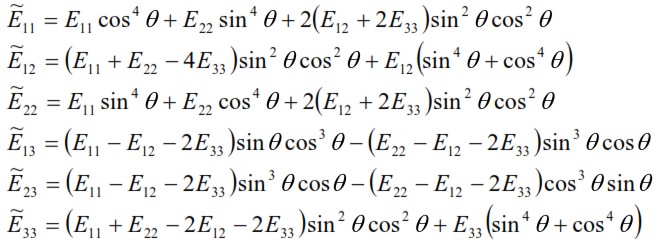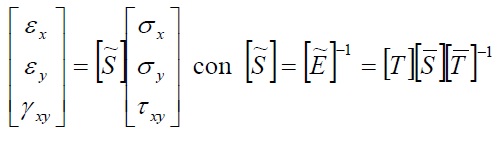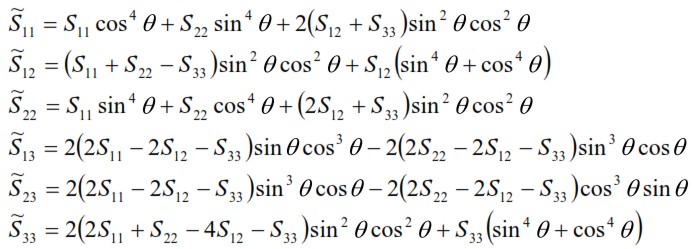1 . Analysis of the Orthotropic Lamina
1.1. Introduction
1.2. Hooke’s Law
1.3. Relationships between elastic constants and Matrix of Elasticity
1.4. Matrix of elasticity
2. Classical theory of Laminates
2.1. Introduction
2.2. Basic Formulas
2.3. Laminate stiffener matrix
2.4. Calculation of Stress and deformation
2.5. Thermal Stress
2.6. Calculation of Elastic Constants
The matrices of elasticity allow to write the stress-strain relationships in the main reference of the laminax. If we consider an arbitrary cartesian system reference the relations between tensions and deformations become more complex, the matrix of elasticity are with all the elements different from zero.
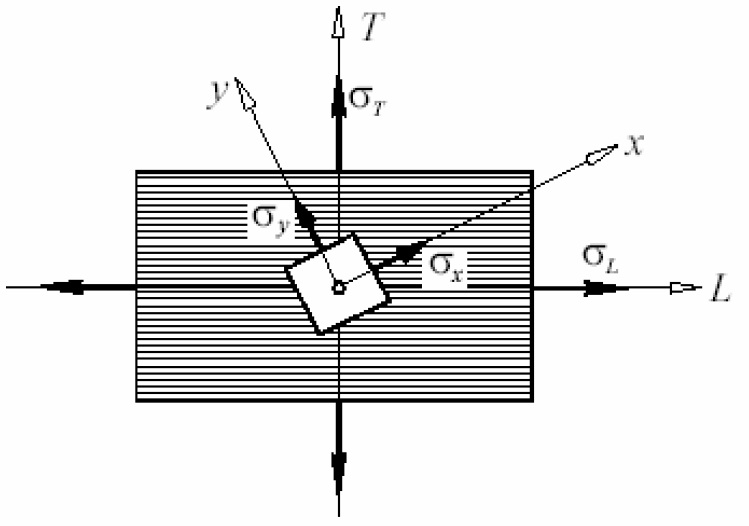
The matrices of elasticity and inverse elasticity in a generic reference Cartesian forming a generic angle with the main reference, can be obtained considering the equations of transformation of the state of stress and strain in the neighborhood of the point. From the general relations for stresses and strains:
Such relationships derived from simple geometrical and equilibrium considerations are valid for isotropic and anisotropic materials. With reference to the Figure 4 we have in particular:
which in matrix form can be written as:
[T] it the rotation matrix given by:
Using (37-38) is finally possible to write from (9-10) the corresponding relations valid in a generic Cartesian reference. Indicating with [Ē] and the matrix which is obtained from the matrix [E] (relative to the axes of the natural material) by simply replacing the term GLT with 2 GLT, for the matrix of elasticity we have:
Dividing by two terms in the third column of the matrix [T]-1 and denoting by [T] the matrix obtained, the equation (40) can be written in compact form:
By inverting (41) we obtain immediately the general relationship between strains and tensions involving the inverse matrix of elasticity in a generic Cartesian reference:
Doing the products shown to the right of the matrix (41) and (48) it is easy to verify that the matrices of elasticity and inverted elasticity are full in the generic reference. This makes it more complex in practice the application of Hooke’s law to switch from the stresses to deformation or vice versa.
For this reason, in practice, Hooke’s law is usually applied after reduction of deformations and stresses in the main reference material using the general relations of transformation of the stress state and deformation in the neighborhood of the point (Eq. 35-26).